In the world of physics and thermodynamics, few equations are as fundamental and widely applicable as q=mc∆t. This simple yet powerful formula lies at the heart of numerous scientific and engineering processes, playing a crucial role in our understanding of heat transfer and energy exchange. In this comprehensive guide, we’ll delve deep into the intricacies of q=mc∆t, exploring its origins, components, applications, and significance in various fields.
Read Impressive Guide to sketch:jgr9lxsmgmg= drawing ideas Unlock Creativity
Whether you’re a student, researcher, or simply curious about the fundamental laws that govern our universe, this article will provide you with a thorough understanding of q=mc∆t. We’ll break down each element of the equation, discuss its practical implications, and examine how it relates to other important concepts in thermodynamics and beyond.
What is q=mc∆t?
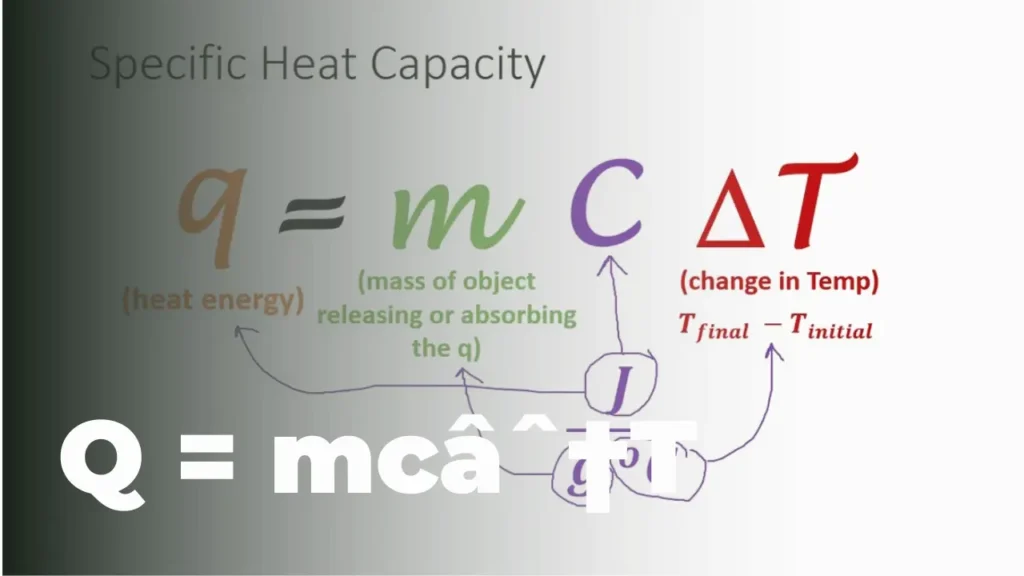
At its core, q=mc∆t is an equation that describes the relationship between heat energy, mass, specific heat capacity, and temperature change. Let’s break down each component of this formula:
- q: represents the amount of heat energy transferred to or from a system
- m: stands for the mass of the substance or object in question
- c: denotes the specific heat capacity of the material
- ∆t: represents the change in temperature
The equation q=mc∆t tells us that the heat energy transferred (q) is equal to the product of the mass (m), specific heat capacity (c), and the change in temperature (∆t). This seemingly simple relationship has far-reaching implications in various fields of science and engineering.
The History and Development of q=mc∆t
To truly appreciate the significance of q=mc∆t, it’s essential to understand its historical context and the scientists who contributed to its development. This equation is closely tied to the broader field of thermodynamics, which emerged during the Industrial Revolution in the 19th century.
Let’s Explore Top Connections Hints Today
Early Thermodynamics
The foundations of thermodynamics were laid by scientists such as James Prescott Joule, Rudolf Clausius, and William Thomson (Lord Kelvin). Their work on heat, energy, and temperature paved the way for more specific relationships like q=mc∆t.
The Contribution of James Prescott Joule
James Prescott Joule, an English physicist, made significant contributions to the understanding of heat and energy. His experiments on the mechanical equivalent of heat were crucial in establishing the connection between mechanical work and heat energy, which is fundamental to the concept behind q=mc∆t.
Let’s Master drawing:cul23ybyzfm= basketball: Tips & Techniques
Formalization of q=mc∆t
While the exact origin of the formula q=mc∆t is not attributed to a single scientist, it emerged as a natural consequence of the work done by various researchers in the field of thermodynamics. The equation represents a distillation of numerous experiments and observations, capturing the essence of heat transfer in a simple, elegant form.
Breaking Down the Components of q=mc∆t
To gain a deeper understanding of this equation, let’s examine each component of the equation in detail:
Heat Energy (q)
In the context of q=mc∆t, q represents the amount of heat energy transferred to or from a system. Heat energy is a form of energy that flows from a warmer object to a cooler one, causing a change in temperature. The unit of heat energy is typically expressed in joules (J) in the International System of Units (SI).
Mass (m)
The mass (m) in q=mc∆t refers to the amount of matter in the substance or object under consideration. Mass is typically measured in kilograms (kg) in the SI system. It’s important to note that mass remains constant regardless of temperature changes, making it a reliable factor in heat transfer calculations.
Specific Heat Capacity (c)
Specific heat capacity (c) is a crucial property in the q=mc∆t equation. It represents the amount of heat energy required to raise the temperature of one unit mass of a substance by one degree Celsius (or Kelvin). Specific heat capacity is usually expressed in units of J/(kg·K) or J/(kg·°C).
Different materials have different specific heat capacities, which explains why some substances heat up or cool down more quickly than others when exposed to the same amount of heat energy. For example, water has a relatively high specific heat capacity, which is why it takes longer to heat up or cool down compared to many other substances.
Temperature Change (∆t)
The ∆t in q=mc∆t represents the change in temperature of the substance or object. It’s calculated by subtracting the initial temperature from the final temperature. Temperature change can be measured in degrees Celsius (°C) or Kelvin (K), as long as the unit is consistent with the specific heat capacity used.
Everything You Need to Know about Soap2Day
The Significance of q=mc∆t in Thermodynamics
The equation q=mc∆t plays a central role in thermodynamics, the branch of physics that deals with heat, temperature, and their relation to energy and work. Let’s explore why this formula is so important in the field:
Quantifying Heat Transfer
One of the primary applications of q=mc∆t is in quantifying heat transfer between objects or systems. By knowing the mass, specific heat capacity, and temperature change, scientists and engineers can accurately calculate the amount of heat energy transferred in various processes.
Energy Conservation
The equation q=mc∆t is closely tied to the principle of energy conservation. It helps us understand how energy is transferred and transformed in thermal processes, ensuring that the total energy in a closed system remains constant.
Thermal Equilibrium
q=mc∆t is instrumental in predicting when two objects or substances will reach thermal equilibrium. By applying the equation to both objects and considering the heat transfer between them, we can determine the final temperature when they reach equilibrium.
Calorimetry
Calorimetry, the science of measuring heat transfer during chemical or physical processes, relies heavily on q=mc∆t. Calorimeters use this principle to determine the heat released or absorbed during reactions, phase changes, or other thermal events.
Practical Applications of q=mc∆t
This equation finds applications in numerous fields, from everyday situations to complex industrial processes. Let’s explore some of these practical applications:
HVAC Systems
Heating, Ventilation, and Air Conditioning (HVAC) systems use the principles behind this equation to calculate the energy required to heat or cool spaces. Engineers use this equation to design efficient climate control systems for buildings, vehicles, and other enclosed spaces.
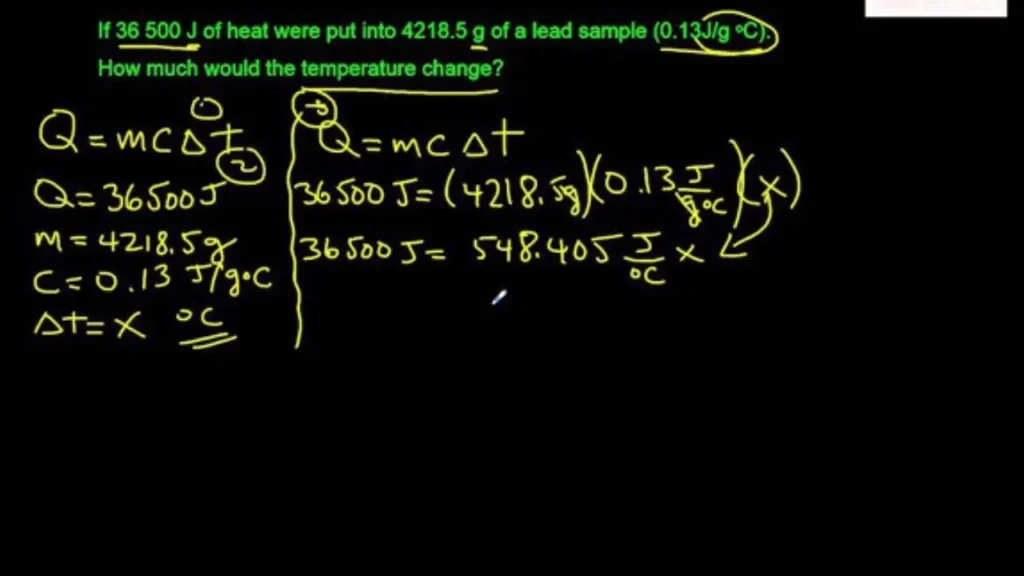
Cooking and Food Science
In the culinary world, this equation helps chefs and food scientists understand how different ingredients and cooking methods affect the heating and cooling of food. This knowledge is crucial for developing recipes, designing cooking equipment, and ensuring food safety.
Industrial Processes
Many industrial processes involve heating or cooling materials. q=mc∆t is used to calculate the energy requirements for these processes, helping optimize efficiency and reduce costs. Examples include metal processing, chemical manufacturing, and pharmaceutical production.
Environmental Science
Environmental scientists use q=mc∆t to study heat transfer in natural systems. This includes understanding the thermal properties of bodies of water, soil, and the atmosphere, which is crucial for climate modeling and predicting environmental changes.
Automotive Engineering
In automotive engineering, this equation is applied in the design of cooling systems for engines, as well as in the development of climate control systems for vehicle interiors. It helps engineers calculate the heat transfer rates and energy requirements for these systems.
Your Guide to Financial Tech News Nasdaq FintechZoom
Medical Applications
The principles of q=mc∆t are used in various medical applications, such as designing incubators for premature infants, developing thermal therapies, and understanding the body’s thermoregulation processes.
Advanced Concepts Related to q=mc∆t
While this equation is a fundamental equation, it’s connected to several advanced concepts in thermodynamics and related fields. Let’s explore some of these advanced topics:
Latent Heat and Phase Changes
When substances undergo phase changes (e.g., melting, vaporization), they absorb or release heat without changing temperature. This is known as latent heat. While q=mc∆t doesn’t directly account for latent heat, it’s often used in conjunction with latent heat calculations to describe complete thermal processes.
Heat Capacity vs. Specific Heat Capacity
Heat capacity (C) is related to specific heat capacity (c) but refers to the amount of heat required to raise the temperature of an entire object or system by one degree, rather than per unit mass. The relationship is expressed as C = mc, where m is the total mass of the object.
Read A Comprehensive Guide moving:wkyidcuim8w= gif wallpaper
Temperature-Dependent Specific Heat Capacity
In some cases, the specific heat capacity of a substance can vary with temperature. For more precise calculations, especially over large temperature ranges, this variation needs to be taken into account, leading to more complex forms of the q=mc∆t equation.
Thermal Conductivity and Heat Transfer Rates
While q=mc∆t describes the amount of heat transferred, it doesn’t account for the rate of heat transfer. Thermal conductivity, which measures a material’s ability to conduct heat, plays a crucial role in determining how quickly heat is transferred in real-world situations.
Entropy and the Second Law of Thermodynamics
The concept of entropy, which is closely related to the second law of thermodynamics, adds another dimension to our understanding of heat transfer. While q=mc∆t doesn’t directly involve entropy, it’s important to consider entropy changes in more comprehensive analyses of thermal systems.
Unlocking The Power Four Digits To Memorize NYT
Common Misconceptions About q=mc∆t
Despite its apparent simplicity, there are several common misconceptions about this equation that are worth addressing:
Misconception 1: q=mc∆t Applies to All Heat Transfer Scenarios
While this equation is widely applicable, it’s not suitable for all heat transfer scenarios. For instance, it doesn’t account for heat transfer during phase changes or in situations where the specific heat capacity varies significantly with temperature.
Misconception 2: The Equation Always Yields Positive Values
It’s important to note that q can be positive or negative, depending on whether heat is added to or removed from the system. A positive q indicates heat being added, while a negative q represents heat being removed.
Misconception 3: q=mc∆t Can Be Used for Non-Thermal Energy Transfer
The equation specifically deals with heat energy transfer. It cannot be directly applied to other forms of energy transfer, such as mechanical work or electrical energy.
Misconception 4: The Equation Accounts for Heat Loss to the Environment
q=mc∆t assumes an isolated system where all the heat energy is transferred to or from the object in question. In reality, some heat is often lost to the environment, which needs to be accounted for in precise calculations.

Solving Problems Using q=mc∆t
To truly master the application of this equation, it’s essential to practice solving problems. Let’s walk through some example problems to illustrate how to use this equation effectively:
Problem 1: Heating Water
Calculate the amount of heat energy required to raise the temperature of 2 kg of water from 20°C to 80°C. (The specific heat capacity of water is approximately 4,186 J/(kg·°C))
Solution:
- Identify the given information:
- m = 2 kg
- c = 4,186 J/(kg·°C)
- ∆t = 80°C – 20°C = 60°C
- Apply the q=mc∆t equation: q = (2 kg) * (4,186 J/(kg·°C)) * (60°C) q = 502,320 J or approximately 502.32 kJ
Problem 2: Cooling a Metal Object
A 500 g aluminum block is cooled from 100°C to 25°C. How much heat energy is removed? (The specific heat capacity of aluminum is approximately 897 J/(kg·°C))
Solution:
- Identify the given information:
- m = 500 g = 0.5 kg
- c = 897 J/(kg·°C)
- ∆t = 25°C – 100°C = -75°C (note the negative value as the temperature decreases)
- Apply the q=mc∆t equation: q = (0.5 kg) * (897 J/(kg·°C)) * (-75°C) q = -33,637.5 J or approximately -33.64 kJ
The negative value indicates that heat is being removed from the aluminum block.
Problem 3: Determining Specific Heat Capacity
An unknown metal object with a mass of 200 g absorbs 1,500 J of heat energy, causing its temperature to rise from 22°C to 35°C. Calculate its specific heat capacity.
Solution:
- Identify the given information:
- m = 200 g = 0.2 kg
- q = 1,500 J
- ∆t = 35°C – 22°C = 13°C
- Rearrange the q=mc∆t equation to solve for c: c = q / (m * ∆t)
- Substitute the values: c = 1,500 J / (0.2 kg * 13°C) c = 576.92 J/(kg·°C)
This specific heat capacity is closest to that of zinc, which is approximately 388 J/(kg·°C). The difference could be due to impurities in the metal or measurement uncertainties.
q=mc∆t in Different Units and Systems
While we’ve primarily discussed q=mc∆t using SI units, it’s important to understand how to work with this equation in different unit systems. Let’s explore some common variations:
Imperial Units
In the imperial system, heat is often measured in British Thermal Units (BTU), mass in pounds (lb), and temperature in degrees Fahrenheit (°F). The specific heat capacity is then expressed in BTU/(lb·°F). The equation remains q=mc∆t, but care must be taken to ensure unit consistency.
Calories and Kilocalories
In some contexts, especially in food science and nutrition, heat energy is measured in calories (cal) or kilocalories (kcal). When using these units, the specific heat capacity is expressed in cal/(g·°C) or kcal/(kg·°C).
Temperature in Kelvin
When working with absolute temperature scales like Kelvin, the equation remains unchanged. However, it’s crucial to remember that ∆t in Kelvin is numerically equal to ∆t in Celsius, making conversions unnecessary when only the temperature change is considered.
All You Need to Know about Google Local Guide Program
The Limitations of q=mc∆t
While this equation is a powerful and widely applicable equation, it’s important to understand its limitations:
Non-Constant Specific Heat Capacity
For some materials, the specific heat capacity can vary significantly with temperature. In such cases, using an average value or integrating over the temperature range may be necessary for more accurate results.
Phase Changes
q=mc∆t doesn’t account for the latent heat absorbed or released during phase changes. When a substance changes phase (e.g., from solid to liquid), additional equations are needed to describe the heat transfer accurately.
Non-Uniform Heating
The equation assumes uniform heating throughout the substance. In reality, temperature gradients can develop within materials, especially in large objects or rapid heating scenarios.
Heat Loss to the Environment
q=mc∆t assumes an isolated system where all heat energy is transferred to or from the object in question. In practice, some heat is often lost to the surrounding environment, which needs to be accounted for in precise calculations.
Complex Systems
For systems involving multiple components or complex heat transfer mechanisms (e.g., radiation, convection), q=mc∆t alone may not be sufficient to describe the entire process accurately.
q=mc∆t in Education and Research
This equation plays a significant role in both education and research across various scientific disciplines:
In Physics Education
q=mc∆t is often one of the first equations students encounter when studying thermodynamics. It serves as a foundation for understanding more complex concepts in heat transfer and energy exchange.
In Chemistry
Chemists use q=mc∆t extensively in calorimetry experiments to measure the heat released or absorbed during chemical reactions. This helps in determining reaction enthalpies and understanding the energetics of chemical processes.
In Engineering
Engineering students across various disciplines, including mechanical, chemical, and environmental engineering, use this equation as a fundamental tool for designing systems involving heat transfer. The equation is crucial for calculations in thermal management, energy efficiency, and process optimization.
In Materials Science
Researchers in materials science use q=mc∆t to study the thermal properties of new materials. Understanding how different materials respond to heat is essential for developing advanced materials for applications ranging from electronics cooling to aerospace engineering.
Read about Prince Narula Digital PayPal Revolutionizing Online Payments
In Earth Sciences
Geologists and climatologists apply q=mc∆t in studying thermal processes within the Earth and in the atmosphere. The equation helps in modeling heat flow in the Earth’s crust, ocean currents, and atmospheric phenomena.
Advanced Applications of q=mc∆t
While q=mc∆t is a fundamental equation, its principles extend to more complex applications in various fields:
Thermal Energy Storage
In renewable energy systems, thermal energy storage often relies on the principles of q=mc∆t. Engineers use the equation to design efficient storage systems, such as molten salt tanks in concentrated solar power plants or phase change materials in building insulation.
Cryogenics
The field of cryogenics, which deals with very low temperatures, applies q=mc∆t in designing systems for cooling and maintaining extremely cold temperatures. This is crucial in applications like superconductivity research and the storage of liquefied gases.
Thermal Management in Electronics
As electronic devices become more powerful and compact, effective thermal management is crucial. Engineers use q=mc∆t to design cooling systems that prevent overheating in computers, smartphones, and other electronic devices.
Biomedical Engineering
In biomedical applications, q=mc∆t is used in the development of thermal therapies, such as hyperthermia treatment for cancer. It’s also applied in the design of temperature-controlled medical devices and in understanding the body’s thermoregulation processes.
q=mc∆t and Energy Efficiency
The equation q=mc∆t plays a significant role in efforts to improve energy efficiency across various sectors:
Building Design
Architects and engineers use the principles of q=mc∆t to design energy-efficient buildings. By understanding how different materials and structures transfer heat, they can optimize insulation, heating, and cooling systems to minimize energy consumption.
Industrial Process Optimization
In manufacturing and chemical processing, q=mc∆t helps engineers optimize heating and cooling processes. By accurately calculating heat transfer, they can design more efficient systems that reduce energy waste and operating costs.
Transportation
The automotive and aerospace industries apply q=mc∆t in designing more fuel-efficient vehicles and aircraft. This includes optimizing engine cooling systems and improving cabin climate control to reduce overall energy consumption.
The Future of q=mc∆t: Emerging Technologies and Research
As technology advances, the application of q=mc∆t continues to evolve. Let’s explore some cutting-edge areas where this fundamental equation is finding new relevance:
Nanotechnology
At the nanoscale, heat transfer behaviors can differ from those at the macro scale. Researchers are investigating how q=mc∆t applies to nanomaterials and nanostructures, potentially leading to breakthroughs in areas like nanoelectronics and nanoscale thermal management.
Quantum Computing
As quantum computers rely on maintaining extremely low temperatures, the principles of q=mc∆t are crucial in designing cooling systems for these advanced machines. Understanding heat transfer at the quantum level presents new challenges and opportunities for researchers.
Artificial Intelligence and Machine Learning
AI and machine learning algorithms are being developed to optimize complex thermal systems. These advanced computational methods can use q=mc∆t as a foundational principle to predict and optimize heat transfer in scenarios too complex for traditional analytical approaches.
Advanced Materials
The development of new materials with unique thermal properties, such as high-temperature superconductors or thermal metamaterials, relies on a deep understanding of heat transfer principles, including q=mc∆t.
Interdisciplinary Applications of q=mc∆t
The versatility of q=mc∆t extends beyond traditional scientific and engineering fields. Let’s explore some interdisciplinary applications:
Forensic Science
Forensic scientists use the principles of q=mc∆t in analyzing crime scenes. For example, understanding how quickly a body cools (algor mortis) can help determine the time of death in criminal investigations.
Art Conservation
Conservators apply heat transfer principles, including q=mc∆t, in preserving and restoring artworks. This knowledge helps in controlling the environmental conditions for delicate artifacts and in applying heat-based restoration techniques.
Sports Science
In sports like winter Olympics events or Formula 1 racing, understanding heat transfer is crucial. q=mc∆t principles are applied in designing equipment, optimizing performance, and ensuring athlete safety in extreme temperature conditions.
Food Technology
Food scientists and technologists use q=mc∆t in developing new food processing and preservation techniques. This includes optimizing cooking processes, designing food packaging, and ensuring food safety during storage and transportation.
Teaching q=mc∆t: Strategies for Educators
For educators teaching q=mc∆t, here are some effective strategies to help students grasp this fundamental concept:
Hands-on Experiments
Conducting simple experiments, such as heating water or cooling metal objects, can help students visualize the relationship between heat, mass, and temperature change.
Real-world Applications
Connecting q=mc∆t to everyday experiences, like cooking or weather patterns, can make the concept more relatable and engaging for students.
Interactive Simulations
Using computer simulations or online interactive tools can help students explore how changing different variables affects heat transfer.
Problem-solving Practice
Providing a variety of problem-solving exercises, from simple calculations to complex scenarios, can help reinforce understanding and develop critical thinking skills.
Common Errors and Pitfalls in Using q=mc∆t
When working with q=mc∆t, it’s important to be aware of common errors and pitfalls:
Unit Consistency
One of the most frequent errors is inconsistent use of units. Ensure that all units are compatible and conversions are done correctly.
Sign Convention
Forgetting to account for whether heat is being added to or removed from a system can lead to errors. Remember that heat added is typically positive, while heat removed is negative.
Neglecting Heat Loss
In real-world scenarios, some heat is often lost to the surroundings. Failing to account for this can lead to inaccurate results.
Assuming Constant Specific Heat Capacity
For some materials and over large temperature ranges, the specific heat capacity can vary. Using an average value or integrating over the temperature range may be necessary for more accurate results.

q=mc∆t in the Context of Climate Change
The equation q=mc∆t plays a significant role in understanding and addressing climate change:
Ocean Heat Capacity
The high heat capacity of water, as described by q=mc∆t, explains why oceans can absorb vast amounts of heat, acting as a buffer against rapid temperature changes but also storing heat that can have long-term effects on climate.
Atmospheric Warming
q=mc∆t helps climatologists model how increased greenhouse gases affect the atmosphere’s ability to retain heat, leading to global warming.
Climate Modeling
Complex climate models use q=mc∆t as one of the fundamental equations to simulate heat transfer between the atmosphere, oceans, and land masses.
Conclusion
The equation q=mc∆t, despite its simplicity, is a powerful tool that underpins our understanding of heat transfer and energy exchange. From its historical development to its wide-ranging applications in science, engineering, and beyond, q=mc∆t continues to be a cornerstone of thermodynamics.
As we’ve explored in this comprehensive guide, q=mc∆t is not just a formula to be memorized, but a concept that helps us understand and interact with the world around us. Whether you’re a student beginning your journey in thermodynamics, a professional engineer designing the next generation of energy-efficient systems, or simply someone curious about the fundamental laws that govern our universe, a deep understanding of q=mc∆t is invaluable.
As technology advances and new challenges emerge, the principles encapsulated in q=mc∆t will continue to be relevant, adapting to new contexts and driving innovation across various fields. By mastering this fundamental equation, we open doors to a deeper understanding of energy, matter, and the intricate thermal processes that shape our world.



